This course is on Mondays 10-11.45, Wednesdays 13.15-15.00, and Thursdays 15.00-16.45.
Location is MVH-12 on Mondays and Thursdays, MVF-21 on Wednesdays.

The topics are as follows:
1. Algebras, sigma algebras, measures, and outer measures.
2. Completion of a measure, creating a measure from an outer measure, and pre-measures.
3. Pre-measure extension theorem and metric outer measures.
4. Metric outer measures.
5. Canonical metric outer measures and Hausdorff measure.
6. Hausdorff dimension.
7. Self-similarity and Hausdorff dimension.
8. Similitudes, Hausdor ff and Lebesgue measures, and Urysohn's Lemma,
9. Similitudes and iterated function systems.
10. The canonical invariant measure of an IFS fractal.
11. The Hausdorff dimension of an IFS fractal and an introduction to complex dynamics.
Notes on the fundamentals of complex analysis (auf Deutsch)
12. Complex dynamics on the disk, plane, and sphere.
13. Fixed points.
14. Conformal conjugation at attracting and repelling fixed points.
15. Super attracting fixed points, irrationally neutral fixed points, and rational iteration.
16. The Fatou and Julia sets of rational functions.
17. Julia sets of rational functions: properties and fractal nature.
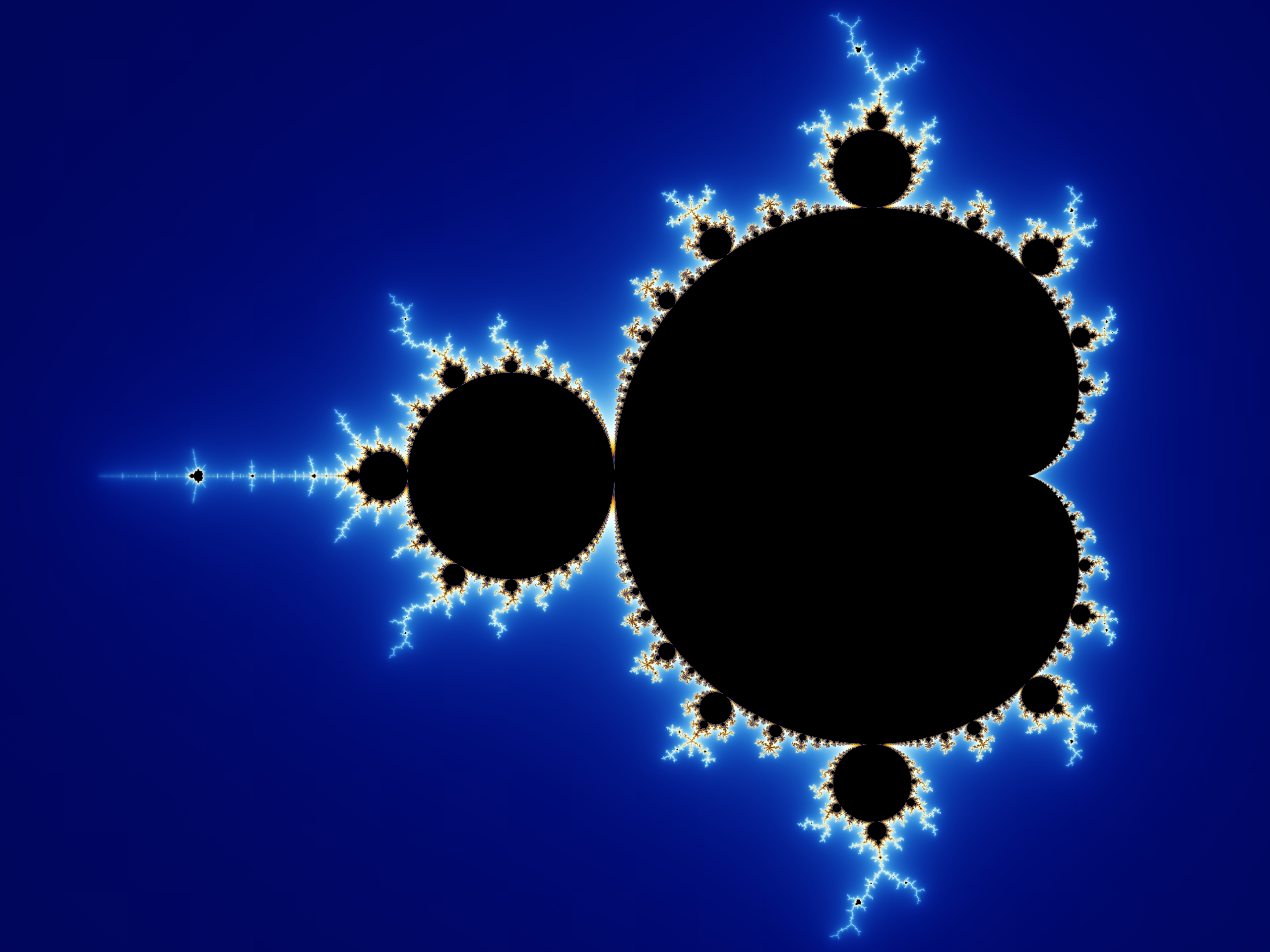
18. The Mandelbrot set.
Lecture Notes
Research Project Ideas
Written Exam
Lecture notes which include an extensive collection of exercises shall be posted after each lecture.
Examination shall be one of the following (your choice): (A) Research project and presentation (can be done in a group or individually), (B) Oral exam, (C) Written exam.